

A cuboctal polycube is a polycube whose cells form the shape of a cuboctahedron.
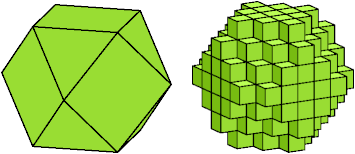
The smallest cuboctal polycube whose volume is a multiple of 5 is the cuboctal polycube with height 7, shown above on the right. It has 195 cells, so it can be tiled with 39 pentacubes.
Bryce Herdt has pointed out that one can define cuboctal polycubes
in which the diagonals of the square
faces are joined at the edge
of the shape:

I call such cuboctal polycubes heavy, and cuboctal polycubes such as the green one above light. Heavy cuboctal polycubes must be fairly large to have their number of cells be divisible by 5. The smallest such polycube has 720 cells. The red polycube shown above has 93 cells.
Here I show which pairs of pentacubes can tile the green polycube shown above, using at least one copy of each pentacube. A prime mark (′) after a letter denotes a mirror image. For example, S′ is the mirror image of S. To see a tiling, click on the corresponding entry in the table below. Missing entries indicate unsolved cases.
The A and F pentacubes can each tile this polycube alone. To see such tilings, click on the corresponding index link in the table. This polycube is the smallest known full-symmetry oddity for the A and F pentacubes.
If you solve an unsolved case, please write.
See also:
| A | B | E | E′ | F | G | G′ | H | H′ | I | J | J′ | K | L | M | N | P | Q | R | R′ | S | S′ | T | U | V | W | X | Y | Z | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | |||||||
| B | @ | @ | @ | @ | @ | – | – | – | @ | – | – | – | – | – | – | – | – | – | – | – | – | ||||||||
| E | @ | @ | @ | @ | @ | @ | × | – | – | – | – | @ | @ | @ | – | – | – | – | – | – | – | – | – | – | – | – | |||
| F | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | @ | ||||||||||
| G | × | – | – | × | – | – | – | – | × | – | – | – | – | – | × | × | – | × | – | – | × | – | – | ||||||
| H | – | – | – | – | @ | – | @ | – | – | @ | – | – | – | – | – | – | – | – | @ | – | – | ||||||||
| I | @ | – | × | × | @ | × | × | – | × | × | × | × | × | × | × | × | |||||||||||||
| J | – | – | – | @ | – | – | – | – | @ | – | – | – | – | – | – | – | – | – | |||||||||||
| K | – | @ | – | – | – | – | × | × | × | – | – | × | – | × | |||||||||||||||
| L | @ | – | – | – | – | × | × | × | – | – | × | × | – | ||||||||||||||||
| M | @ | @ | @ | @ | @ | × | @ | @ | @ | × | – | @ | |||||||||||||||||
| N | – | – | – | – | – | – | – | – | – | – | @ | ||||||||||||||||||
| P | – | – | – | – | – | – | – | – | – | – | |||||||||||||||||||
| Q | – | × | – | × | – | – | – | – | – | ||||||||||||||||||||
| R | – | – | – | – | – | @ | – | @ | – | – | |||||||||||||||||||
| S | × | × | × | × | – | × | × | × | |||||||||||||||||||||
| T | × | × | – | × | × | × | |||||||||||||||||||||||
| U | × | – | × | × | × | ||||||||||||||||||||||||
| V | – | × | – | × | |||||||||||||||||||||||||
| W | @ | – | – | ||||||||||||||||||||||||||
| X | × | × | |||||||||||||||||||||||||||
| Y | × | ||||||||||||||||||||||||||||
| Z | |||||||||||||||||||||||||||||
Last revised 2024-03-19.