
The January 2008 issue of Erich Friedman's Math Magic defined a frame as a square polyomino with a centered square hole. The problem was to find the frame with least area that could be tiled with a given polyomino.
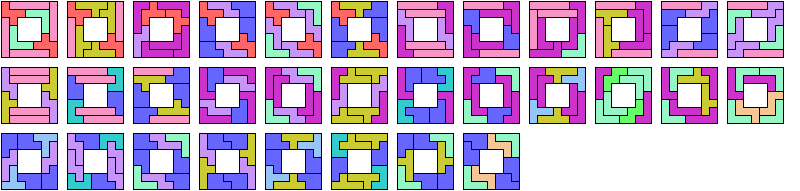
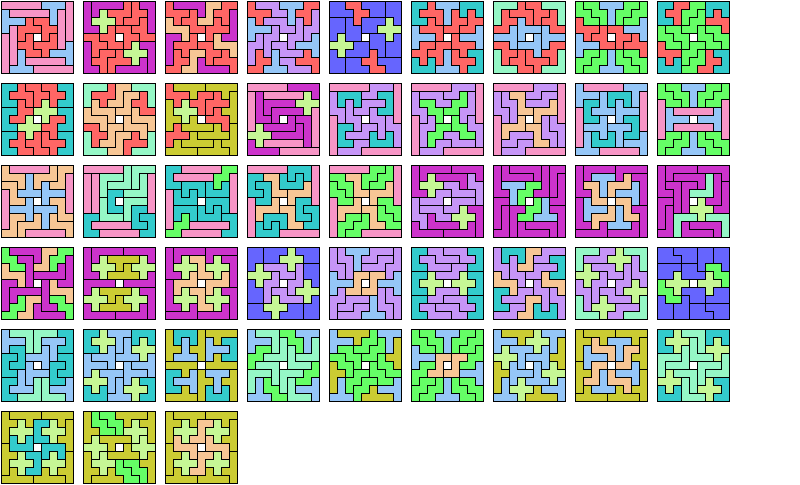
Here I study the related problem of finding the smallest frame that can be tiled with copies of three pentominoes.
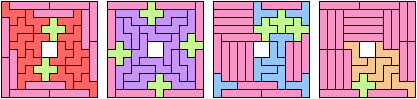
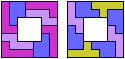
Carl Schwenke and Johann Schwenke improved on two of my solutions.
See also Two-Pentomino Square Frames.

| F I L | 12 | F I N | 12 | F I P | 12 | F I T | 16 | F I U | 12 | F I V | 8 | F I W | 12 | F I X | 28 | F I Y | 8 | F I Z | 24 |
| F L N | 8 | F L P | 12 | F L T | 12 | F L U | 12 | F L V | 12 | F L W | 12 | F L X | 16 | F L Y | 12 | F L Z | 16 | F N P | 8 |
| F N T | 16 | F N U | 12 | F N V | 8 | F N W | ? | F N X | ? | F N Y | 12 | F N Z | ? | F P T | 12 | F P U | 12 | F P V | 12 |
| F P W | 12 | F P X | 16 | F P Y | 8 | F P Z | 12 | F T U | 16 | F T V | 16 | F T W | 16 | F T X | ? | F T Y | 12 | F T Z | ? |
| F U V | 12 | F U W | 16 | F U X | 16 | F U Y | 12 | F U Z | 12 | F V W | 24 | F V X | 24 | F V Y | 12 | F V Z | 16 | F W X | ? |
| F W Y | 12 | F W Z | ? | F X Y | 16 | F X Z | ? | F Y Z | 12 | I L N | 8 | I L P | 8 | I L T | 12 | I L U | 12 | I L V | 8 |
| I L W | 12 | I L X | 16 | I L Y | 8 | I L Z | 12 | I N P | 8 | I N T | 12 | I N U | 16 | I N V | 8 | I N W | 16 | I N X | 28 |
| I N Y | 8 | I N Z | 16 | I P T | 12 | I P U | 8 | I P V | 12 | I P W | 12 | I P X | 12 | I P Y | 8 | I P Z | 12 | I T U | 16 |
| I T V | 12 | I T W | 16 | I T X | 28 | I T Y | 12 | I T Z | 16 | I U V | 16 | I U W | 16 | I U X | 12 | I U Y | 12 | I U Z | 16 |
| I V W | 24 | I V X | 40 | I V Y | 12 | I V Z | 12 | I W X | 48 | I W Y | 12 | I W Z | 16 | I X Y | 24 | I X Z | 28 | I Y Z | 12 |
| L N P | 8 | L N T | 12 | L N U | 12 | L N V | 8 | L N W | 12 | L N X | 16 | L N Y | 8 | L N Z | 12 | L P T | 12 | L P U | 8 |
| L P V | 8 | L P W | 12 | L P X | 12 | L P Y | 12 | L P Z | 12 | L T U | 12 | L T V | 12 | L T W | 16 | L T X | 24 | L T Y | 8 |
| L T Z | 16 | L U V | 12 | L U W | 12 | L U X | 12 | L U Y | 12 | L U Z | 12 | L V W | 8 | L V X | 16 | L V Y | 8 | L V Z | 8 |
| L W X | 24 | L W Y | 12 | L W Z | 16 | L X Y | 16 | L X Z | 16 | L Y Z | 12 | N P T | 8 | N P U | 8 | N P V | 8 | N P W | 12 |
| N P X | 16 | N P Y | 8 | N P Z | 12 | N T U | 12 | N T V | 12 | N T W | 12 | N T X | 24 | N T Y | 12 | N T Z | 16 | N U V | 12 |
| N U W | 12 | N U X | 16 | N U Y | 12 | N U Z | 16 | N V W | 12 | N V X | 16 | N V Y | 12 | N V Z | 12 | N W X | ? | N W Y | 12 |
| N W Z | ? | N X Y | 12 | N X Z | ? | N Y Z | 12 | P T U | 12 | P T V | 12 | P T W | 12 | P T X | 12 | P T Y | 8 | P T Z | 12 |
| P U V | 12 | P U W | 12 | P U X | 12 | P U Y | 8 | P U Z | 12 | P V W | 12 | P V X | 12 | P V Y | 8 | P V Z | 8 | P W X | 16 |
| P W Y | 12 | P W Z | 12 | P X Y | 12 | P X Z | 12 | P Y Z | 12 | T U V | 16 | T U W | 12 | T U X | 16 | T U Y | 16 | T U Z | 24 |
| T V W | 16 | T V X | ? | T V Y | 12 | T V Z | 12 | T W X | 24 | T W Y | 16 | T W Z | 16 | T X Y | 16 | T X Z | ? | T Y Z | 16 |
| U V W | 40 | U V X | 16 | U V Y | 12 | U V Z | 12 | U W X | 24 | U W Y | 12 | U W Z | ? | U X Y | 16 | U X Z | 48 | U Y Z | 12 |
| V W X | 56 | V W Y | 12 | V W Z | 12 | V X Y | 12 | V X Z | 48 | V Y Z | 12 | W X Y | 16 | W X Z | ? | W Y Z | 12 | X Y Z | 16 |









Last revised 2024-02-27.